NOTES:
What are some implications of the how the Ring is laid out?
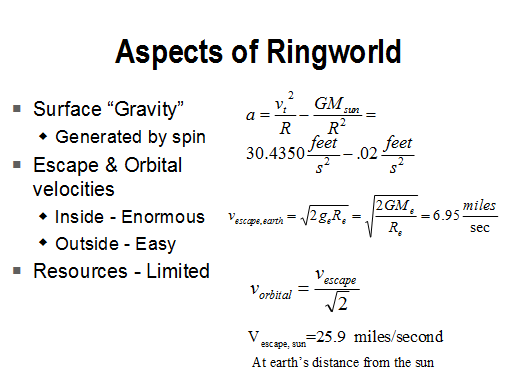
There is surface "gravity" caused by the Ring's rotation and somewhat attenuated by the sun's gravity - in fact the surface gravity is almost identical to Earth's.
However, the cost of gaining standard gravity with rotation is that it's not easy to get off the Ring. Orbital velocity near earth's surface is only about 5 miles per second and escape velocity is only about 7 miles a second - velocities that can be achieved with chemical rockets. On Ringworld, however, any object lofted away from the surface must achieve a velocity of 740 miles/second in the right direction or the velocity it already has by being on the Ringworld will slam the object into the Ringworld's surface with great force. On the other hand, if there were an way to get to the outside of the Ring, simply letting go would give you enough velocity to escape the star's gravity entirely. For that matter, of course, even landing on the Ringworld would be tricky, since matching the surface's velocity would be required and that velocity is far above the natural velocity of an object orbiting the Ringworld's sun at the Ringworld's radius. In the novel, the heroes crash-land, but this is not recommended for anyone without an impervious stasis field. Catty Nebulart pointed out via email that escape from Ringworld (or landing on the outside of the Ring) can be thought about by considering escape from the Ring as transfer from an orbit that momentarily has a radial velocity of zero and a tangential velocity of 740 miles/second to a circular orbit that needs to have a tangential velocity of 18 miles per second (or the reverse process for landing on the outside of the Ring); the need to add or subtract a huge amount of velocity very quickly is obviously a huge challenge for conventional rockets.
Exercise for the student of materials - how strong is the Ringworld's material? Very strong indeed to survive the tension induced by the tremendous spin, but can you calculate how strong it would have to be? How would you assemble the Ringworld? Can you calculate the trajectory of a rocket launching from the inside of Ringworld, relative to the Ringworld? Relative to inertial space? Keep all that in mind, as I go through the math for what happens when someone on the inside of the Ring aims a rocket directly towards the Ring's sun; even though the best approach is to add velocity tangentially, not radially, it's still interesting to see how the rocket's trajectory deviates from the trajectory that would occur on a planet (where it does make sense to aim your rockets up!).
Catty Nebulart also corrected a typo on this page - I had the velocity to escape from the Sun's gravity (at the Earth's distance from the Sun) as 18.3 miles per second, which is actually the Earth's orbital speed.
