NOTES
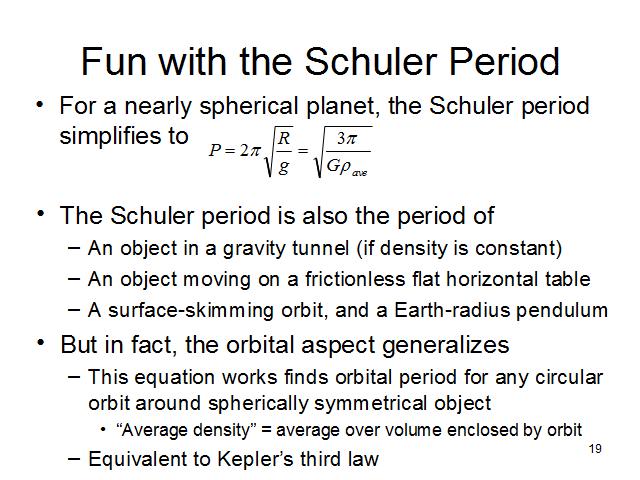
Kepler's third law is that the orbital period of a planet is directly proportional to the 3/2th power of the semi-major axis of its orbit - and this equation for the Schuler period in terms of average density turns out to be that law in disguise for circular orbits (average density decreases with the cube of radius, but the equation here has a square root; putting those factors together produces the 3/2-power relationship between radius and period). This is a way to get a rough measure of G, the gravitational constant, or to measure average density of a newly discovered planet (just orbit an small object around it and measure the period).
