NOTES
Before we go on, let's talk a bit about directions...
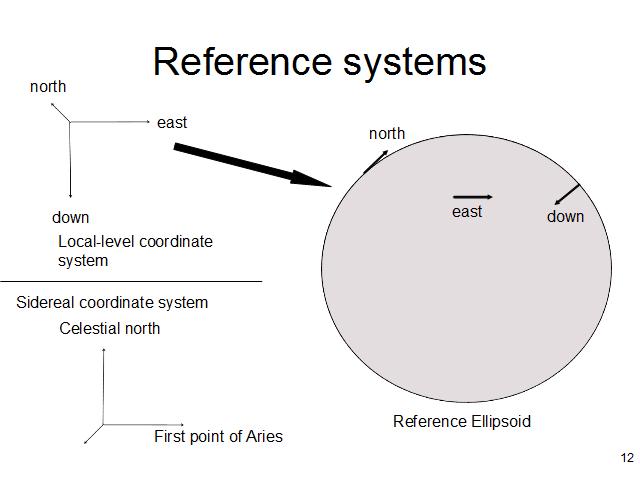
There are any number of reference systems that can be used for inertial navigation but there are two of them that are particularly useful for this discussion - the locally level earth-fixed system and the sidereal system. The locally level system is tied to the Earth - two axes define a plane perpendicular to the reference ellipsoid (the ellipsoid that best fits the earth) and the third axis points down - the direction that a plumb bob points); each point on the Earth's surface has a different version of the locally level frame. Meanwhile the sidereal coordinate frame is defined with reference to the fixed stars - this is the frame in which the gyroscopes stay pointed, and relative to which the accelerometers measure acceleration. The direction of the buoyant force is always up in the locally level frame, and varies continuously in the sidereal frame, changing as the Earth rotates and the vehicle moves across the surface of the earth. In the upcoming illustrations, I'll use a rectangle tangent to the surface of the earth to symbolize the inertial system's knowledge of where the local level "down" is - just remember that errors in the orientation of the local level frame are equivalent to errors in position; every possible orientation of the local frame relative to the inertial frame is right for some position on Earth - just not necessarily the position you're actually at.
Here's where I use my Hoberman sphere toy and some three-dimensional coordinate axes (made of Q-tips) to demonstrate the fixed sidereal frame and the changes in local level coordinate frame as the earth rotates and the vehicle moves.
